Subtle forces yield profound effects on heavy element bonding

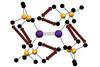
Calculations on dispersion forces shed light on interactions between heavier main group elements