Looking back – and forwards – at the unusual structures
The award of the 2011 chemistry Nobel prize to Dan Shechtman for his discovery of quasicrystals in the early 1980s was something of a surprise to many seasoned Nobel-watchers. Quasicrystals are one of those concepts that almost no non-scientists have heard about, and to many practising scientists is something on the edge of their awareness. Maybe they learned about it in a short section of a crystallography or solid-state physics course – or maybe not at all.

That’s certainly not to say the award was a shock, nor that it wasn’t well-warranted. After all, Shechtman and his collaborators had forced the re-writing of the textbook definition of a crystal, surely one of the most fundamental concepts in science. Understandably, most mainstream media accounts of the prize made a lot of this, and in particular focused on the apparent feud between Shechtman and Linus Pauling. The double-Nobel laureate and legendary structural chemist – one of the few chemists with an almost household name level of fame – had repeatedly dismissed Shechtman, even saying ‘There are no such things as quasicrystals – just quasi-scientists’.
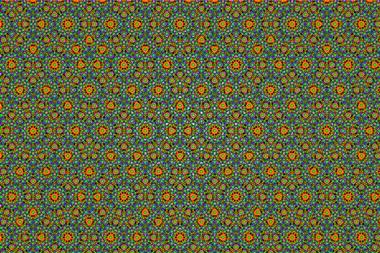
But much of the wider coverage glossed over the science behind the prize. That’s fairly understandable, given how difficult it is to get your head around the concept of three-dimensional order that is somehow not periodic: with rotational symmetry, but no translational. Fortunately for picture editors everywhere, beautiful two-dimensional examples exist to illustrate our articles and do a great job of getting across the ‘order without periodicity’ conundrum.
I was lucky enough to hear Shechtman speak at a conference in 2013, where he pointed out that quasicrystalline materials are not particularly unstable or even rare. After the talk, I asked him how it had taken so long for them to be discovered, bearing in mind mineralogy and crystallography’s long histories. He explained that many of the crystals are small, and so it had taken transmission electron microscopy – and a professional working on the instrument – to discover the fivefold symmetry.
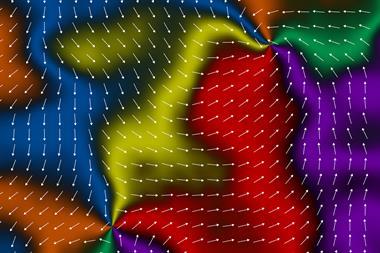
For all their fundamental importance and mind-bending interest, however, almost no quasicrystalline materials have found an application. Anna Demming’s feature on p48 looks at some of the recent work in this area, and at some other ‘not quite crystalline as we know it’ materials. It’s great to see that a group at the University of California in Berkeley, US, has already made use of ‘the hat’ shape, an aperiodic tile discovered in 2022 that can tesselate a surface without translational symmetry – a kind of two-dimensional quasicrystal. They made a polymer composite out of 5mm hat shapes, and the irregular boundaries meant it was stronger than a regular periodic structure would be.
For all their fundamental importance and mind-bending interest, however, almost no quasicrystalline materials have found an application. Anna Demming’s feature looks at some of the recent work in this area, and at some other ‘not quite crystalline as we know it’ materials. It’s great to see that a group at the University of California in Berkeley, US, has already made use of ‘the hat’ shape, an aperiodic tile discovered in 2022 that can tesselate a surface without translational symmetry – a kind of two-dimensional quasicrystal. They made a polymer composite out of 5mm hat shapes, and the irregular boundaries meant it was stronger than a regular periodic structure would be.
While not every discovery needs to be exploited, of course, it will be fascinating to see whether the unusual structures that aperiodic crystals produce result in unusual and interesting properties too.













No comments yet